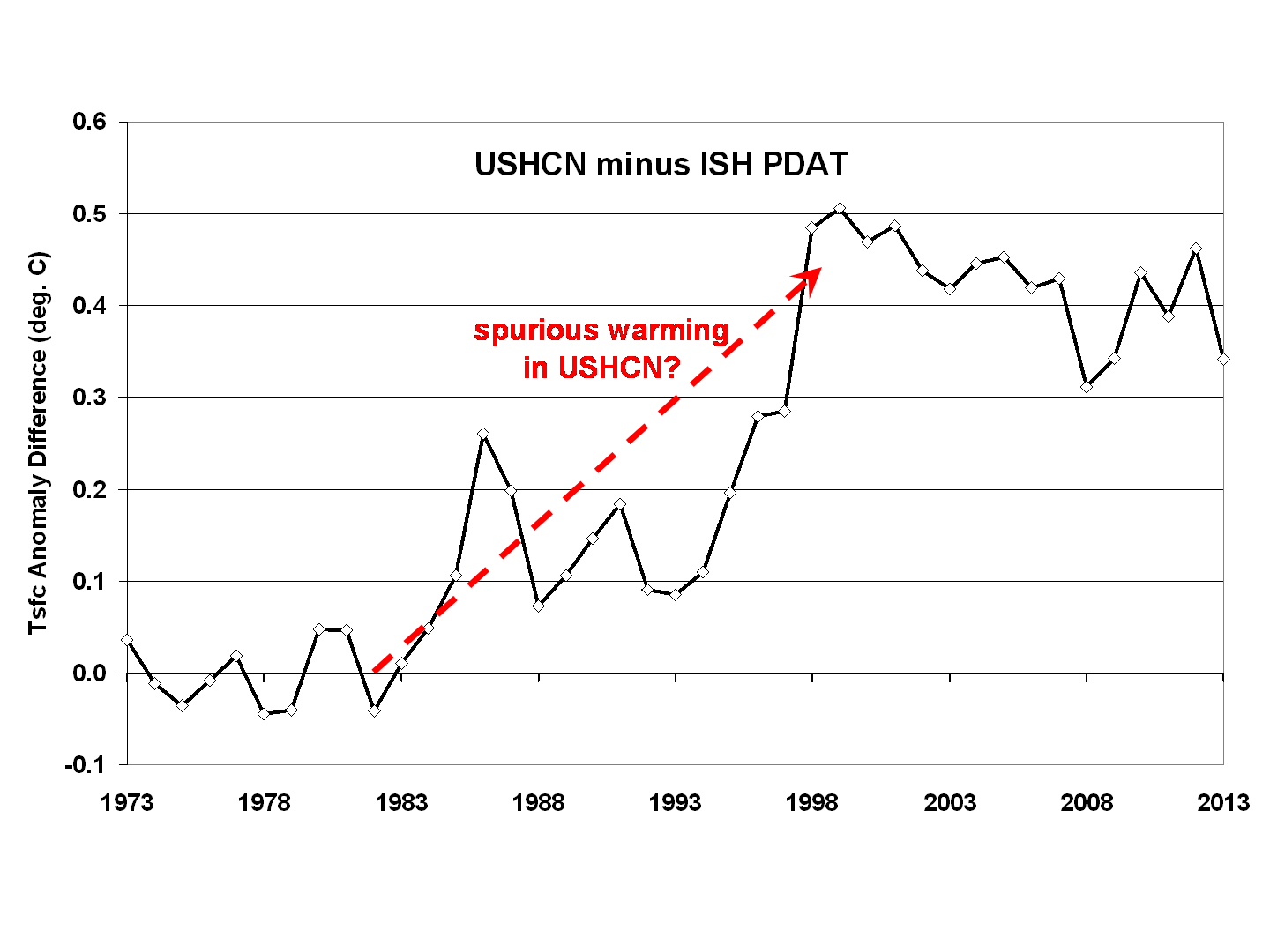
Home/BlogSteve Goddard recently posted some results from his analysis of the official U.S. surface temperatures (USHCN, from NOAA) suggesting spurious warming occurring around 1998. I also showed evidence of this back in 2012.
Steve’s post reminded me that it’s been over a year since I’ve updated the U.S.-average Integrated Surface Hourly (ISH) temperature data, using my Population Density Adjusted Temperature (PDAT) algorithm that corrects for changing urban heat island (UHI) effects. This is still an unpublished method, and so should be considered more of a sanity check on the official NOAA USHCN product. But it does support Steve’s contention that there’s something funny going on in the USHCN data.
One of the big differences between my ISH PDAT dataset and the official NOAA products is that mine is based upon about 270 stations which monitor hourly temperatures, from which I compute a daily average temperature from the observations at 00, 06, 12, and 18 UTC. This avoids time-of-observation problems associated with computing daily averages from maximum and minimum temperatures, as is done in the USHCN dataset.
Secondly, my dataset only starts in 1973 because that’s the first year with reasonably complete coverage of the U.S. with the hourly observation sites (not the cooperative observer sites which dominate the USHCN dataset which are more numerous but report daily max and min temperatures).
Thirdly, my adjustment for UHI effects is more straightforward than the NOAA homogenization procedure, which I consider rather “opaque”. I believe the NOAA methodology is prone to warming rural sites to match urban sites, rather than cooling the urban sites to match the rural sites. I can’t prove this because, as I said, the homogenization methodology is, well, opaque. In the U.S., my population density adjustment ends up subtracting off an average of about 0.1 deg. C/decade from the temperature trends, but that varies for each station depending upon the change in population density over time. A good place to start for a description of the population density adjustment to temperatures is here. (I now use a constant population density vs. temperature curve for all areas except the Pacific Northwest and the southwest U.S., which show no obvious average warming effects with population density.)
The yearly temperature anomalies for 1973-2013 show that, for the contiguous 48 states, the USHCN Tmax+Tmin observations indicate considerably more warming than the 4x/day temperature observations adjusted for local population density changes (dashed curve fits are 2nd order polynomials):
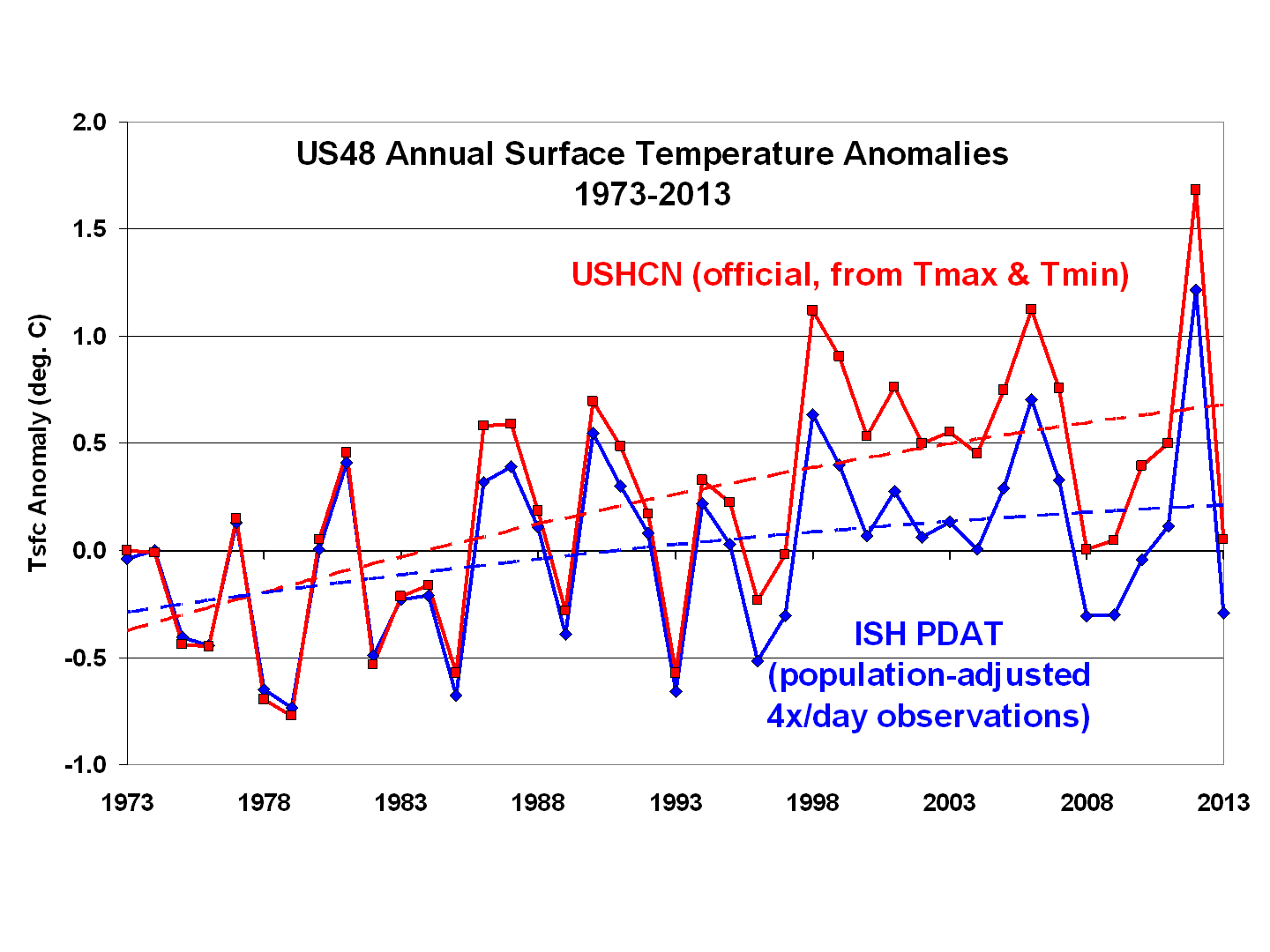
A difference plot of the 2 datasets, as I showed almost 2 years ago, reveals the biggest discrepancy occurs around 1998:
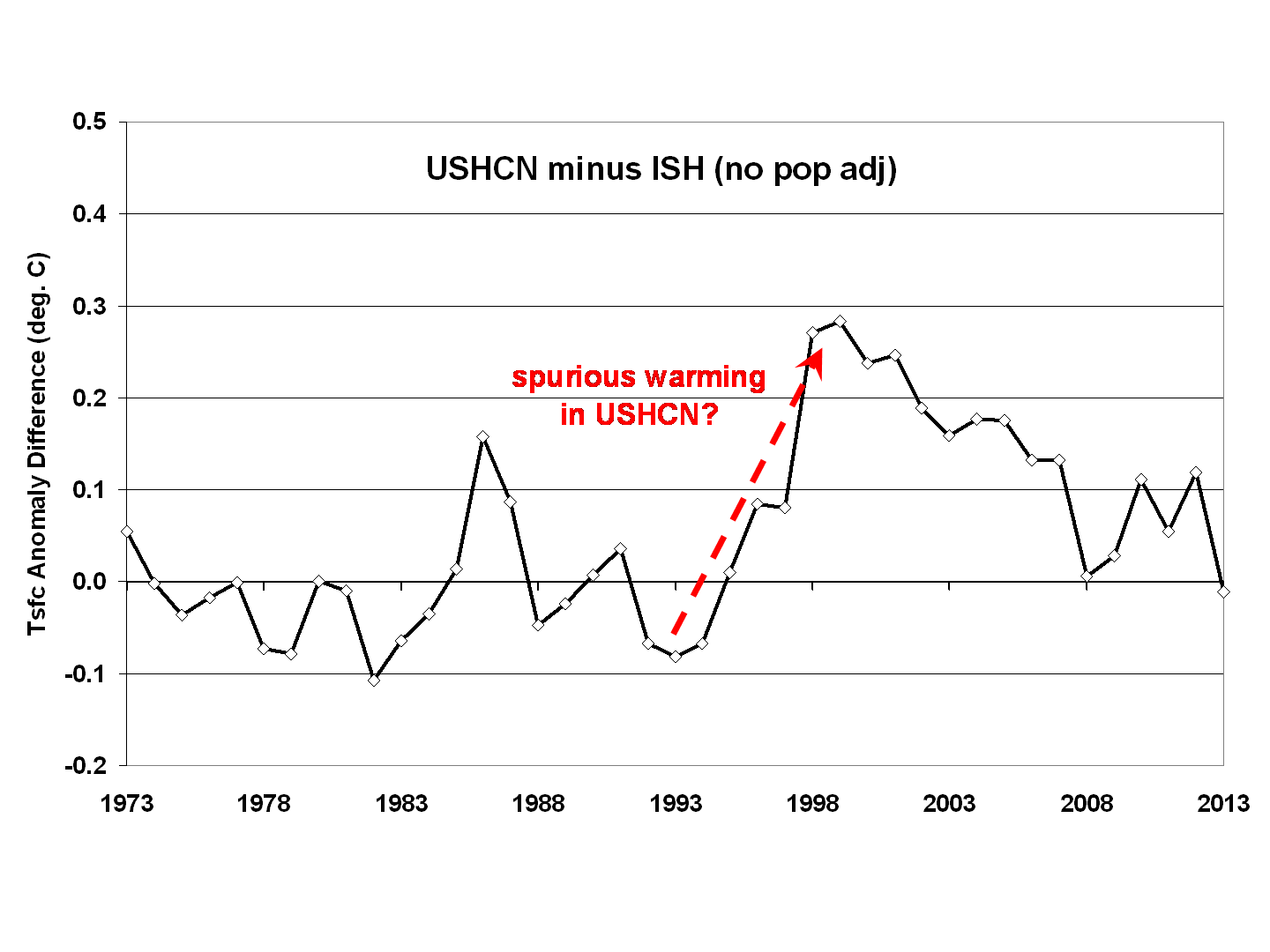
I don’t know all of the sources of these discrepancies, which partly remain even if I *don’t* do a population density adjustment to my dataset:
At some point I need to update the population density adjustment, which originally relied on only 1990 and 2000 census data, which I extrapolated forward and backward in time. Now that NASA/SEDAC has population density estimates up to the present, this would provide some improvement to the adjustments for urban heat island effects.
Clearly, adjustments to surface temperature data are at least as large as the global warming signal being sought. Until a transparent analysis of the USHCN methodology is carried out, and alternative methods and temperature datasets are tested, I can’t bring myself to believe any U.S. government pronouncements regarding record warm temperatures.
ADDENDUM
I see that my previous posts don’t really provide the info needed for those interested in how I’m doing the temperature trend adjustments from changes in population density over time. Here’s the regression relationship I am using:
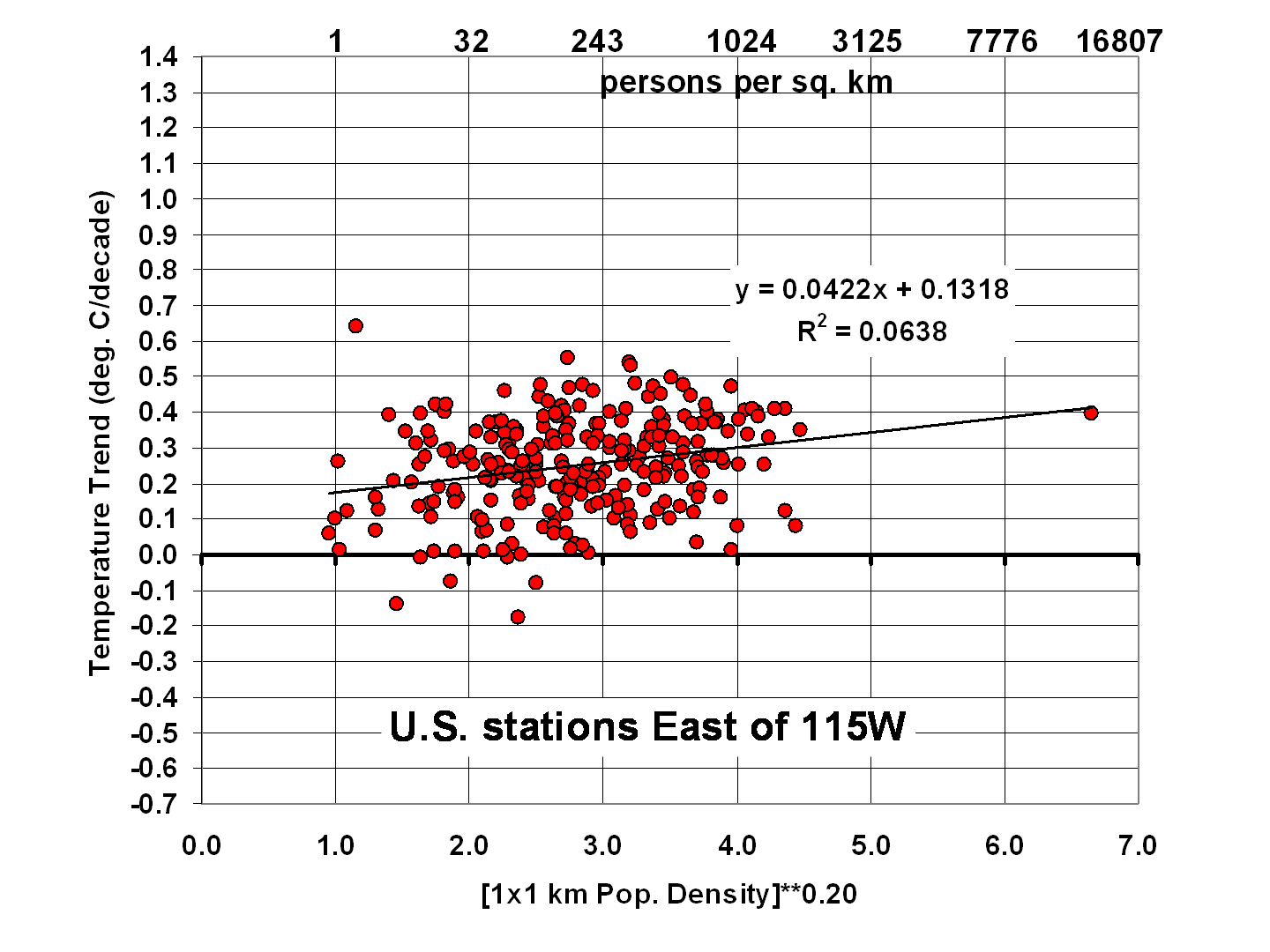
For each station east of 115W, I adjust its temperature anomaly time series using the 0.0422 regression coefficient applied to the change in station location population density (to the 0.2 power) between 1990 and 2000 (extrapolated back to 1973, and forward to 2013). The adjustment starts at zero in 1973, then decreases the trend linearly with time if the population went up, or decreases increases the trend with time if the population went down. The 0.2 power factor is consistent with previous studies that showed the strongest UHI effects occur early in population growth, then level off at higher population densities.
Since you are addressing various ways to massage the data, please give us your tak eon this paper that states that the current record underestimates the real warming trend. If you are a real scientist you of course wd know what you put here, unreviewed is in fact worth nothing. This is a refereed article, give us your critique, then try to get yours published.
http://onlinelibrary.wiley.com/doi/10.1002/qj.2297/abstract
That article does not apply to the U.S., which is the subject of my post. Nice try, though.
No it applies to the whole world and that is what we are talking about isn’t it? GLOBAL warming? So it does include the USA, but alos the rest of the world. You can pick and choose as you please but that is not what a real scientist does
That paper claims that certain undersampled regions probably warmed more than the more-sampled regions…which might be true for the Arctic, prob not true for most of the southern hemisphere. (In any event, it proves nothing about the cause of warming anyway.)
But that undersampling problem is only one source of error, and if the UHI effect is contaminating GLOBAL data (as there is also published evidence to support) then those UNDERSAMPLED areas would be not warming as fast as we think, either.
In other words, the UHI effect trumps the incomplete sampling effect. Everyone knows urban areas are warmer than rural areas (there are MANY published papers with measurements), and yet no one has shown that the urban temperature trends in the current datasets have been adjusted downward to compensate for the effect.
Who is this Parsons twit, anyway? All I ever see from him is attacks and insults combined with falsified graphs and nonsensical “data sets”.
I don’t know where you learnt statistics. But there appears to be no linear or other relationship between population density and temperature.
You may want to look at latitude or some other measure with population density to give a meaningful result.
Any use of such a poor curve fit will only result in a worse answer.
But what only really matters are the temperatures for the globe.
I am glad about the extreme cold for the U.S.A in that it weakens the global warming theory, due to the fact the cold is due to a more meridional jet stream pattern which is tied to solar activity(ozone concentration changes and distributions),and not the lessening amounts of Arctic Sea Ice due to global warming, as is the AGW theory view of matters as being responsible for this type of pattern.
In a side note the lower then normal amounts of Arctic Sea Ice can be tied to the AMO being in it’s warm phase.
CLIMATE ARGUMENT
My argument is two fold in that mainstream science does not appreciate just how variable the sun can be, and does not accept the many solar/climate SECONDARY connections that are a result of this variability.
In addition the sun has yet to approach the criteria I have called for on a constant basis (which I have sent on many previous post) to have A MAJOR climate impact thus far.
In addition solar activity from 1850-2005 has been to constant to manifest itself into any big climate impacts. This however could change this decade as solar activity has been running much below normal post 2005 , despite the recent maximum of solar cycle 24. This maximum should end soon and time will tell how quiet the sun becomes going forward.
The other climate areas which are not given enough attention to when it comes to solving the climate puzzle in my view are the earth’s magnetic field strength, past history (Maunder Minimum) which shows solar/climate connections, Milankovitch cycles combined with current solar activity to get an overall impact, the beginning state of the climate which can give an entirely different climatic result even if the same climate forces are applied.
I agree that the global average is what we should be most interested in. But when I’ve tried to analyze surface temperature data from other regions, the coverage is so sparse and quality is so poor that I am reluctant to believe any of the results as being representative of the globe (or even of the northern hemisphere).
how convenient. Maybe it is your analysis that is of such low quality. You don’t get to subjectively select out what you don’t like- real scientist don’t do that
thanks for the knowledgeable critique. You are obviously very discerning regarding what is good science. 😉
There is an old Chinese saying: “He is most wise who knows what he does not know.”
A good scientist is able to distinguish between quality data and questionable data.
The IPCC is not able to do so. IPCC personnel are so blinded by political agendas that they do not do what good scientists should do. Again and again, we see science playing second fiddle to politics in the IPCC.
You need to do a bit more extensive reading B Parsons. Here in Australia our BOM (Bureau of Meteorology), which is a loud supporter of AGW, has also fiddled with older high temperature land based data, on the basis that the older thermometers were not accurate enough. Of course as elsewhere, apparently, that earlier high temperature data has been reduced. Also no reasonable attempt has been made to account for UHI.
There is plenty of evidence that higher maximum temperatures occurred in many parts of Australia back in the 1880s than any recent supposedly “record” high temperatures.
Also heat waves, it is claimed, are becoming more prevalent. Of course the BOM has reduced “heat wave” criteria down to 35C for several days. For some of us who have been around for a while that is hardly even getting warm. Certainly nothing like the “heat waves” we experienced in the middle of the 20th century.
Interestingly, the very same thing happened ‘across the ditch’ here in New Zealand in 2009. The National Institute of Water & Atmospheric Research (NIWA – then headed by Dr Jim Salinger formerly of the CRU in East Anglia)had systematically adjusted data to create a warming trend.
http://nzclimatescience.net/images/PDFs/global_warming_nz2.pdf
And people wonder why there is mistrust of climate scientists?
B Parsons
Perhaps you could deign to stoop to examine the temperature evidence at Darwin Zero and wax eloquent on the quality, reliability and predictive value of such official peer reviewed results – and compare that to the analysis provided!
Adelaide has a very good temp data set going all the way back to the 1800’s. It was started by one Charles Todd (of Todd river fame in Alice Springs) He also started the overland telegraph and at each telegraph station he built a weather station and teh Australian weather service was born. These data sets show many hot years right up to 1906 unfortunately/conveniently the BOM data sets begin in 1910.
I have just finished an exhaustive debate here in Adelaide with someone who seems similar to Mr parsons, at one point early in this debate this person called me a denier. As the days wore on i realised just how little he actually knew. It got to the point were i knew it was a complete waste of time when i had to explain to him water has the states, Liquid, Solid and Gas as in GHG. This poor fool had no idea water had a gaseous state and when you consider this piece of information lies at the very heart of the AGW theory then you will begin to understand why it was exhaustive.
The moral is that even though Mr parson’s or people like him may sound knowledgeable it is more than likely the he is as dumb (re basic physics)as a box of hammers so dont waste too much time on him.
regards
Crakar
Dr. Spencer is the most real climate scientist you will ever come across. The most objective person I have seen thus far. Very fair and will post all points of view on the climate.
Then Salvatore you must be the 2nd “most real” one out there…because man, you really look outside the box.
http://climate.nasa.gov/nasa_role/science
Solar irradiance variation is already included in climate change models!!
the variation is on the scale of 0.1% of the suns output.
Irradiance at Earths surface = 1350 W/m2
1350*0.001=1.35
therefore using this variation the Irradiance reaching the Earth’s surface could drop to:
1350-1.35 = 1348.5 W/m2
WOW!!
now can you please turn off your broken record…do some other REAL research
Salvatore is not referring to simple TSI but rather to the way that solar variability can be amplified by other interactions with the Earth system such as changes in solar wavelengths or particles affecting ozone amounts at different heights and latitudes in the stratosphere which is an area that I am interested in.
It is those who refer solely to TSI who need to replace their broken record.
Wheat prices were correlated to the solar cycle a long time ago – I find it hard to imagine that a plant is so sensitive that its productivity is significantly affected by a 0.1% change in the suns output. It seems to me there must be something else going on as well. How are those effects included in the models?
Wheat crops are affected by the latitudinal shift of the rain bearing winds above the regions where they are grown.
Those shifts are not at all explained by a simple change of 0.1% in TSI.
Hence my focus on those latitudinal shifts and I have come to the conclusion that the gradient of tropopause height between equator and poles is all important.
That is heavily influence by the ozone content of the stratosphere because that is what created the tropopause in the first place by reversing the temperature gradient above the tropopause.
The changes in the mix of wavelengths and particles from the sun between periods of high and low activity must be affecting ozone amounts in the stratosphere differently between equator and poles so as to affect that gradient of tropopause height.
In turn that affects global cloudiness and the amount of solar energy able to enter the oceans to fuel the climate system.
In due course I think that is going to become as obvious as plate tectonic theory has now become.
You are so correct. I hope the satellite data will finally resolve this problem going forward.
Note , the more meridional jet stream pattern can also be linked to the sea surface temperature patterns in the North Pacific Ocean. The warm pool off Western Canada, in that part of the globe.
Dr. Roy: Don’t you think the distribution of global anomalies is more meaningful than just the average? If the antarctic did really warm and the tropics cooled to produce a zero average anomaly the effects would be very different from the reverse. The average of the whole globe is important but not as meaningful as the distribution IMHO.
I think the global average anomaly is the single most important metric…regional anomalies tend to offset one another depending upon circulation patterns. Only in the global average is there no vertical motion, which can profoundly impact temperatures.
Now, that being said, certainly the regional distribution of anomalies tells us something…although climate models aren’t good enough yet to tell us just what those regional differences are telling us.
Those regional differences are the thermostatic mechanisms in action.
Either top down solar effects or bottom up oceanic effects will cause the regional differentials to change.
Such effects are never evenly distributed which is the cause of the subsequent system reaction. Any unevenness leads to temperature and density differentials in the horizontal plane which then leads to motion in the vertical plane.
The convective circulation pattern between regions then changes to try and neutralise the regional solar induced or ocean induced anomalies whether they be towards net warming or net cooling.
The reason is that regional anomaly distributions represent uneven surface heating so one sees temperature and density differentials between parcels of air at the same height.
That inevitably leads to convection as less dense parcels (low pressure systems)must rise above more dense parcels (high pressure systems) against the force of gravity and vice versa.
The result is a remarkably stable system with the baseline system energy content determined by atmospheric mass, gravity and top of atmosphere insolation but with constant variation either side of the mean as the negative system response adjusts the circulation pattern as necessary to negate any forcing element other than mass, gravity or TOA insolation.
On that basis you would get a climate response to GHGs in the form of circulation changes but that would not be discernible compared to solar and oceanic effects.
I am an accountant, and I am fascinated by the sudden break between the 2 datasets in 1998. A change in generally accepted accounting principles causes the same effect when the prior years are not restated to reflect the change in method.
Also, in the late 90’s, at least in my field, there were a lot of system conversions as newer, more powerful software became available. In many instances, due to compatibility issues, data could not be imported directly from the old systems into the new ones.
Large amounts of complex historical data entered by hand, or summarized by time period, sometimes resulted in gross errors that were carried forward until someone had the good sense to ask.
Government agencies are notoriously bad at record retention and system conversions. Health.gov is only the most recent example.
NOAA should provide not only a detailed explanation of their USHCN methodology, but also a log of events surrounding this time period. I would be very interested to see it.
Dr. Spencer, your work is fascinating. Thank you for taking the time to share it.
I suspect NOAA is pretty good at archiving old data…that’s what they do. The problem comes in when they decide exactly how to adjust the data for multiple partially-known problems. That’s where all kinds of arguable decisions are made.
Riki
If you do explore the data, make sure you get the original unadjusted raw data. As Spencer notes above, there are questions as to the current published data. See http://stevengoddard.wordpress.com/2013/01/11/noaa-temperature-fraud-expands-part-1/
For comparison, the AWOS sounds interesting:
You started the graphic in 1973 with USHCN and your model equal zero but if there is a difference that difference should show up back then. Or is your point that the USHCN data is systematically being tampered with (as per Phil Jones admitted for Hadcrut) This is an alternative to the opaque homogenization of the USHCN data not a pleasant thought in the science but given the political environment probably needs to be considered.
using 1973 as the zero point is arbitrary…I’m sure there is spurious warming in the data prior to that. The anomalies in both datasets are relative to 1973-2013, but then I adjusted the 1st 5 years of the USHCN data to match the average anomalies from the ISH data for those 5 years. So, I’m only addressing issues relative to the 1973-2013 period.
Roy Spencer,
“In the U.S., my population density adjustment ends up subtracting off an average of about 0.1 deg. C/decade from the temperature trends,…”
0.1 ° C per decade, interesting.
An old canard which require to be updated but the principle is there:
http://img708.imageshack.us/img708/1363/anomthn.png
“I believe the NOAA methodology is prone to warming rural sites to match urban sites, rather than cooling the urban sites to match the rural sites. I can’t prove this because, as I said, the homogenization methodology is, well, opaque.”
Maybe not so opaque than that. NOAA, like other agencies worldwide, corrects sudden jumps of temperature series, these are pretty easy to spot. The most significant of these corrections are related to changes of sites. These changes of sites generally correspond to an improvement of the environment (less perturbations or UHI) and thus sudden cooling which are corrected. Unfortunately, the gradual warming of the sites is never corrected. See in this regard Hansen et al. 2001.
Having spent a multitude of hours studying the NOAA and GISS methodology, I believe a good description is “opague.” Also, my inquiries have been answered in a less than satisfactory manner. If my students were as evasive as NOAA and GISS have been, I would suspect that they were cheating.
One source of warming is aging of the white surface of instrument shelters. Someone at wattsupwiththat.com said this. And the warming is assumed to be real rather than caused by aging of the instrument shelter.
I consider the loud people at W.U.W.T. to be mostly biased and significantly cherrypicking, but this issue rings true to me.
Non-true warming due to an aging instrument shelter would warm daytime readings and not nighttime readings. Growth of urban heat island effect would warm nighttime and early morning readings more than midday and afternoon readings. Is anyone working on equations to separate these effects?
The r-square for our regression is 0.06. Does this suggest that a different correlation approach should be used? When I do a regression and get those kinds of r-squares and don’t use the regression.
Looking at the regression figure at the bottom it seems as if the slope is mainly determined by the outlier to the far right while all the rest of the points for stations with lower population density is just a big blob with no discernible trend. This method may be a simple way to get a temperature adjustment, but it hardly seems reliable.
My eyeballs are telling me that the far-right datum is not much contamination, despite its extreme position. What I see is that excluding this datum would make the slope a little steeper, and also have a slightly lower correlation coefficient.
I think there needs to be more exploration of effect of growth of urban presence. I think a more accurate equation will be messier than effect = (population density change) ^ a constant. For one thing, people may be changing the size of their urban footprints.
As for my experience in Philadelphia: I think growth of urban effects outside the city have warmed the (largely non-growing) city since the 1970s. I see this in hot summer nights getting hotter, with dewpoints not rising over the decades as much as temperatures did, and decreasing nighttime and dawntime relative humidities.
This article is your most ridiculous example of data mining and massaging that I have read yet.
You have an agenda here Dr. Spencer. Your naivety to the actual science and data are disappointing. Like B. Parsons says you cannot simply pick and choose data because you do not like what it tells you. The BEST project eliminated the possible over exaggeration of the heat island. At least Dr Richard Muller was man enough to come out and admit his hypothesis was wrong.
The thing is we as scientist cannot publish false data because its methodology will be ripped to shreds in peer review. Especially on such an incredibly important issue as this as ground breaking research on global warming hits the most critical and scrutinizing journals. As a enviro. scientist I would love for climate change to not be an issue, but all data and predictions point directly at MAJOR problems. Furthermore, continually pumping this much of anything into our atmosphere and perturbing natural cycles this much is going to have MAJOR impacts, especially when these gases (GHGs) clearly interact with energy on the wavelengths emitted by the earth
Of course greater population density will lead to greater effects of GHGs. More people = more GHGs in atmosphere. Thus more warming. so you don’t like this and take it out of the data?!
That is the poor science Parsons is talking about.
AGENDAS BIAS REAL SCIENCE
@Cupsui,
You seem to think that UHIs’ effects shouldn’t be accounted for in the data and corrected/adjusted. If UHI effect isn’t corrected for (ie., by correcting downward) it would be analogous to a grass placed weather monitoring site being concreted over, and then WOW temperature increase…see “global” warming.
As a statistician, if I know that certain data is unreliable, or of poor quality, or poor methodology I don’t use it. That isn’t cherry picking the data, it’s making sure that it isn’t misused. It is, in fact, very good science.
Hey Cupsui, . is your other ‘post name’ B. Parsons . . . ?
You are acting like the southbound end of a northbound horse.
. .oh, you’re a Global Warming Believer. . .
Now I see, that explains it ALL.
Oh, enjoy replying to me, and making some snarky comment.
No one who sees this will believe you.
No my other post name is not B. Parsons as I’m sure the good Dr. can support by my IP address.
and no I’m not a global warming believer I am a scientist that studies environmental issues. I analyse data and do experiments. I read peer reviewed science on these issues. Peer reviewed science that does not allow falicies (not in respectable journals, where scientific breakthroughs this important are published).
I don’t just use and bend data, which is all that this website addresses, even Dr. Roy Spencer admits himself a lot of the papers he reviews for you to read are above his level of learning.
Its not about believing its about learning…try it. its great!
As a scientist Cupsui you should know that all you have to do is turn your modem off and back on and you can have a different IP address or maybe you were not taught that during your gruelling 3 years of study?
Question….Does Dr Spencer have an agenda or is he simply naive?
Back on topic, do you have information that supports the idea that the land based temp data sets produce accurate information, if so i suggest you divulge that information rather than continuing down the path of insults and character smears.
Of course, human population affects things.
However, the issue here is hotspots of human population and growth thereof, and associated *local* effects, being disproportionately considered in recording of *global* temperature.
The world is not warming as fast as its growing towns, cities, and megopolises.
Cupsui. Hmmm like Parsons you don’t seem to be very informed. Has the population of the US decreased recently? No? Well I leave it to you to discover why its GHG emissions has, so we have been informed, has fallen. Hint power fuel sources.
Here’s another hint. Is there a difference in GHG emissions when there is a decline in industrial activity.
Here’s another interesting line of enquiry that may enlighten even the most trusting CAGW disciple: What proportion of our industrial CO2 emissions, at most, end up in the atmosphere?
What happens to the rest?
Some go into the atmosphere some go into the ocean. But as the temperatures increase the capacity for CO2 to dissolve into the oceans decreases. Therefore oceans and the atmosphere are moving closer to equilibrium. The greater we affect the carbon cycle the larger burden we place on “sinks” and with increasing temps all these “sinks” will begin to emit CO2 as a secondary source.
US has done something about CO2 emissions by turning to fracking. Tell me are there no problems with fracking? Again the potential problems will be left for future generations to clean up. And energy return over energy invested for shale gas is not high. Its a short term fix…another Band-Aid.
No GHG emissions would not decrease immediately with a shift to renewable energies because there is a lag-time. secondary sources will continue to emit. That’s why action now is vital.
Earlier models did not account for aerosols decreasing the effect of warming but now they are accounted for. Thus they have been improved. This is the whole purpose of modeling to give the most accurate interpretations of what may happen in a realistic scenario and then describe the uncertainties involved.
Anything else. This is not simply a statistical problem, which most of you people seem to think. Again it is based on solid science (spectroscopy) that is relatively easy to understand in a basic sense. Try to learn it rather than just playing with numbers. it may help
Cupsui,
Lets start at the begining, if we measure all of the co2 emitted from the earth in one year and then subtract the amount from human activities we will find that 96% of all emissions are from a natural source. I would have thought someone of your training would know this however you dont come across as an envirmental scientist so i thought it best i dont leave anything out. Now that you know what small numbers we are talking about we can now put things into perspective.
The key point here is how much warming will this small increase in CO2 make for without this warming all your doomsday predictions cannot come to fruition. Therefore the first act must be to establish whether the planet is warming or not. We know the planet has not warmed for at least 15 years even Dr Spencer can attest to that through his RSS work.
Obviously we must ensure these measurements are accurate otherwise there is no point in even bothering. If we look at the raw data we see there is no warming or very little warming depending on the data set, Steve Goddard states the USHCN raw data shows a 90 year cooling trend, after adjustments are applied we have a rather large warming trend up until 1998 or so.
Does anyone know what these adjustments are?, are they a negative or positive adjustment, what is the magnitude of the adjustments and most importantly what are the justifications for the adjustments.
To ask these questions is not to be in denial, to ask these questions should not be seen as an affront on what you believe, in fact it is the opposite. You should be asking these questions after all you are a scientist. Once again if you have information that may help Dr Spencer clarify this conundrum then please produce the information.
First point. You were talking about naïve right? 96% this figure seems about right. So we are adding 4% new emissions every year. Some of that will be taken up by soils, sediments and the ocean. Then it will be rereleased. You claim this is a natural release. Can you see the problem here? We are increasing the amount going into the atmosphere, which in turn increases CO2 deposition and also increases re-emissions from these sources. PERTURBATION OF THE CARBON CYCLE! hence why there is a great concentration of CO2 (and methane) in the atmosphere, sediments, soils and the ocean. Proven by ice cores and tree rings and deposited sediments!
“We know that the planet has not warmed for the last 15 years”
Really. So because 1998 was one of (if not) the hottest year on record and now temperatures are consistently nearing that level there has been no warming? What is it 9 of the last 10 years are the hottest on record? Put a STRAIGHT line of best fit through the UAH data set and bob’s your uncle its increasing.
Steve Goddard again is not a scientist. He again is simply playing around with data. His theories are continually debunked and nothing he writes gets peer-reviewed. Is that what you base your “solid” science on? Peer review stops data manipulation and its stops shoddy experimentation and shuts down poor science in general.
The emerging problem stems from people beginning once again to discount scientists when the information they provide does not agree with what politics, big business and peoples way of life in general. It is all well and good when science creates i-phones, I-pads, rocket ships, state of the art weaponry aimed at destroying life, but as soon as incredibly strong evidence for human being adjusting the chemistry of the earth, its atmosphere and heat balance because of our consumption of fossil fuels, industries we have rooted our civilization on then its back to the inquisition days of burning scientists at the stake.
And for the record I am studying a PhD in environmental chemistry focusing on the atmosphere and in particular mercury. Oh which by the way is just another side effect of burning fossil fuels in particular coal
http://www.thestar.com/news/canada/2013/12/12/new_federal_employee_rules_chokes_whistleblowers_say_critics.html
this is becoming common place
@Cupsui, it was only a matter of time before someone tried that nonsense arguement about “muzzling”. For your information, Canada’s Public Service Act has been in existence since the early 1960s and is very specific about public employees giving interviews. They must seek permission from higher authority before making public statements. Why, you might ask – since you obviously know nothign about Canadian laws or proactices and we do NOT subscribe to the US “freedom of speech” interpretations: it is because when a public employee of the Government of Canada makes a public statement it affects national policy. To have a public servant speak incorrectly can cause problems inthe delivery of public services, affect trade agreements, public safety, and even the economy. Scientists are still free to publish their results, to address conferences etc and otherwise make their research known (exceptions being strategic or national security matters).
Let me make it clear for you: NOBODY IS BEING MUZZLED despite claims by some fruitbats and “journalists”. Is that clear enough for you?
“Cupsui says:
January 31, 2014 at 10:08 AM
First point. You were talking about naïve right? 96% this figure seems about right. So we are adding 4% new emissions every year.”
That is 4% of total annual CO2 emissions, which comprise 4% from man-made, and 96% CO2 emissions from natural sources, which shrinks the man-made portion of the “4% new emissions every year” to 0.16% of the total “new emissions every year”, hardly enough to move the flea on the tail that wags the dog.
More: https://www.youtube.com/watch?v=2ROw_cDKwc0 (Presentation by Prof. Murry Salby in Hamburg, on 18 April 2013)
@Bill starling
http://www.thestar.com/opinion/commentary/2013/03/25/closure_of_experimental_lakes_area_part_of_assault_on_science_scharper.html
effective, cheap scientific research project destroyed by the Harper government because the results are not in line with the governments economic policy.
There is your muzzling.
It cost $2million/year to run and $20million to shut down and the Harper govt. claims only the $2million/year in savings. So in 10years the savings would equal out. Unfortunately none of the data is attainable in that time of course.
That is the disgusting behavior of politics muzzling science!
@Cupsui, at least TRY to get the name correct. As for your use of the Star as a reference….one of the most biased paper in Canada is hardly a good reference, and you quote an opinion peice to boot! The project was defunded as it was not producing anything of use. NOT all research is worthy of public funds. Hardly muzzling, even by your extreme standards. But if you love that project so much, kick in some of YOUR money. Stop trying to tell other countries what to do.
Capsui, you said (Jan 27 @ 3:52) “I am not a global warming believer.” Yet you imply a shift to renewable energies is necessary to reduce GHG emissions and “action now is vital.” You later claim “9 of the last 10 years are the hottest on record” and “incredibly strong evidence for human being adjusting the chemistry of the earth, its atmosphere and heat balance because of our consumption of fossil fuels.” This sounds like classic AGW dogma to me.
If you’re still working on your PhD, you’ve learned a lot. You may even get to a point where you know more about mercury in the environment than anyone else. But, hopefully, you won’t get to the place where you think you know it all.
I also hope you mature beyond the arrogance and ignorance manifest in one of your previous paragraphs:
“This is not simply a statistical problem, which most of you people seem to think. Again it is based on solid science (spectroscopy) that is relatively easy to understand in a basic sense. Try to learn it rather than just playing with numbers. it may help”
It isn’t clear what you’re talking about—CO2 sinks, models, global warming, or the subject of this post. Most of us probably thought this post was about measurements and data presentation, not statistics. And there’s nothing scientifically solid about using CO2 absorption/emission spectroscopy as a surrogate for showing any effect of rising CO2 on global temperature. It’s rather like playing with numbers.
check out our neighbouring planets for a couple examples of the effects of CO2 and other GHGs on the atmospheres and temperatures of those planets. If energy re-raditated from the earth interacts with a GHG molecule it is then absorbed and trapped in the atmosphere and not released. Thus more heat stays in the atmosphere?! that forces disturbances in heat balance, rainfall patterns, pressure differences (storms).
And Walter Schnieder you are claiming that humans are not responsible at all for CO2 levels rising? so 4% of emissions are new emissions each year and 4% of those are new anthropogenic emissions? And what percentage of the old emissions are anthropogenic? I really give up on you people. it is impossible to teach knowledge to people who don’t have an open mind to learn. You listen to people who talk about different ways to look at data to derive the information that they want to see. Again peer review does not allow this because poor science, methodology and interpretation is rejected.
Let me guess I am talking to a bunch of baby boomers (or near to it) here. The generation responsible for the largest part of this mess, that in their twilight years does not want to be remembered as the stubborn generation that messed it up for their own greed. Clinging onto power and making the younger generations pay for your retirements and health insurances and ivory back scratchers. Enjoy you Dr Roy Spencers et al. who write the answers you want to hear without even entering the realm of science.
Science is not a pick and choose system, but that is the way it is being used by politicians, big business and baby boomers!! goodbye and thanks for all the fish…oh wait there are none of those left…you ate them all!
Whoa, add impudence to the list.
“If energy re-radiatated from the earth interacts with a GHG molecule it is then absorbed and trapped in the atmosphere and not released.”
Do you know how long a CO2 molecule remains excited after absorption? Compare that with the average time between collisions with other air molecules. How do you quantitate radiation that is trapped, let alone heat? Do you know any peer-reviewed papers that measured how much more heat is trapped by an incremental increase in atmospheric CO2?
“I really give up on you people. it is impossible to teach knowledge to people who don’t have an open mind to learn.”
Is it possible your mind is closed to any facts disturbing your AGW theory?
Hi Dr. Spencer,
being an old Meteosat HAM radio “Fan” (I wrote a DOS software to receive its Low-res imagery in early ’90s and few years later, with some friends, I designed an HRV/HRPT board for the Hi-res imagery) I read with particular interest this research:
ears.nl/user_files/04-Rosema_b.pdf
What is interesting to me it’s that they cleverly used the 10.5-12.5um sensor(they repeatedly wrote “mm”, but it’s surely a misprint), which is CO2 independent and “only slightly [affected] by atmospheric water vapor” to get the temperature anomaly at noon and midnight from 1982 to 2006 for the whole geostationary FOV of the satellite.
Even if they haven’t in my opinion captured the whole outgoing LWIR radiation (which wasn’t their goal of course), I think that this is a better way to establish the land/sea temperature variation than use broadband radiometers affected by the LWIR active gasses.
Note that (if they didn’t have committed some gross evaluation errors which I’m not trained to discover) it seems that using this analysis, the globe showed “a significant cooling” instead of a global warming in those days.
Have a nice day.
Massimo
Neat! The truth will leak out, given enough people looking in enough places.
Very interesting Massimo PORZIO, hmm, the cooling Earth…. http://ears.nl/user_files/04-Rosema_b.pdf .
Thanks for the link.
Personally, I have already ruled out the homogenization adjustments as trash science imprinted in all major manipulated datasets but even after removing these adjustments from HadCRUT you still get the very pronounced ≈62 year cycle of about ±0.25°C that appeared at least post the 1800s. Could this be only due to wetness/dryness fluxes over the years we are seeing there? What that swatch of land in Iraq temperatures as they drained the swamps and dryness set in points out some incredible evidence. Does make you question what is going on.
Hi Wyne,
as said, maybe they did have committed some gross evaluation errors in their paper.
But I found very smart the way they used the narrow band detector to “view” the surface temperature, where the atmospheric “fog” (WV & CO2) doesn’t have almost any effect.
Doing that, they don’t need to correct raw data with modeled factors wider than the anomaly that they want to highlight from the analysis.
Have a great day.
Massimo
Hoops,
missed an “a” in your name… Sorry
Roy, I actually think USHCN looks okay. I think the real problems are in the rest of the world.
Here is my reasoning:
First, take the USHCN monthly anomalies (use the 1981-2010 climatology) from December 1978 to the present. Then take the UAH v 5.6 anomalies for the lower forty eight states. If you are following me so far, the next thing to do is to detrend both of them. Then do a regression with the UAH detrended anomalies as the independent variable, and the USHCN anomalies as the dependent variable. You should get a coefficient for the regression of somewhere between 1.2 and 1.3. If you then *multiply* the raw UAH anomalies by that coefficient, you should find that there is essentially *no* difference between the trends.
So USHCN looks okay. HOWEVER! If you repeat that process with a *global* dataset, you will find that using this same kind of variance adjustment technique indicates substantially lower trends from satellites compared to surface data.
Thanks Dr. Spencer. An interesting article.
I think that when the data has to be adjusted by more than the size of the signal one is trying to extract, the results become so convoluted that you have to know a lot about that signal for the results to be meaningful.
In this way confirmation bias is very possible
Cupsui, the climate models are in a word junk, because they will never have the beginning state of the climate correctly, they will never have complete and enough accurate data, and they can not account for all the different forces which effect the climate at any given time.
Look at their predictions thus far, way off in a word. pathetic .
In regards to my research versus the IPCC , I have no agenda they do. If you choose to believe what they have to say that is fine and time will tell who is correct and who is wrong.
Thanks for the excellent post Dr. Spencer.
The whole debate about AGW reminds me of a debate long ago between the “contagionists” and the “anticontagionists” in regard to the cause of disease. The anticontagionists generally disliked the implications of contagionism — the need for government programs and practices not favored by big business, such as the need to quarantine crews of merchant ships. Thus the anticontagionists promoted objection after objection to the new germ theory of disease.
The debate went on for decades until improvements in instruments and gathering of data showed contagionism the obvious winner. But until the conclusion was obvious, political views informed people’s positions.
Over time, the number of anticontagionists got smaller and smaller, and eventually their view was considered ludicrous. Nowadays, their view seems absurd and we can hardly believe there was even a debate.
Of course the debate had implications beyond science. Many people sickened and died because the anticontagionists provided cover for the business interests that preferred inaction.
History may not repeat, but it often rhymes.
Very poor analogy. To quarantine a few ships is a minor inconvenience in the scheme of things. You might think the same minimum effort is needed to lower carbon emissions. Put up a few hundred thousand wind turbines throughout the world and thousands of square miles of solar panels. And a few minor personal inconveniences on your own part. Would that do it? WRONG! We have a world population that is growing and that wants to live like those of us who have the time and resources to read this blog in a comfortable home. There is no stopping that growth. It will happen no matter how much we want to keep those unlucky(by geography of birth) unfortunates poor. What it would take is a willingness to let the Western wealthy economies crash and a MUCH lower standard of living for all us that live there. It still won’t matter, because China is where the world CO2 emissions are growing.
It is your own lack of insight into what the reality and costs of curbing WORLDWIDE truly would be that parallels your lack of insight into the enormous scam that “renewable energy”, climate change and anthropomorphic global warming is.
But I am sure it gives you a “cozy” feeling to know that you really care and want something done about it. Although I am quite sure that you personally would not want to take the measure that are truly necessary for you to do you fair share. Take a trip to a third world country and tell me if they have the kind of houses that you wish to live in, the shop where you would want to shop, the restaurants you would want to eat in and that list goes on and on.
Do your part right now. Take 1/3rd of your net worth, so you won’t be tempted to buy CO2, which goes into making just about every single possession you won, take that 1/3 of your net worth, burn it in a barrel, and see how that feels.
After you burn 1/3rd of your current and future net worth, then tell me that is just like spending a couple days in quarantine.
Actually, at one time, 40 days in quarantine was common practice, so it was not trivial, and the British empire in particular was anticontagionist. So I think it is a very good analogy. There were also many social implications of contagionism, such as how people viewed tuberculosis. Not surprisingly, anyone with tuberculosis, including some rich and powerful people, did not care for contagionism.
Anyway, long before fossil fuels are exhausted, they will become too expensive, so one way or another we had better start preparing. Fracking notwithstanding, gas is still near $4 a gallon.
In fact, the Energy Return on Investment for a wind turbine is now better than that of domestic oil. The poor of the world already cannot afford a lot of fossil fuels, and the cost of fossil fuels will only go up as demand rises and easily extracted sources are depleted.
Hops,
Another analogy i like to use is the one about that guy who discovered the earth revolves around the sun, upon announcing his discovery he was told to shut up by religious leaders for they feared the information may losen their vice like grip of control on the general populace.
Feel free to use this whenever the need arises.
Note, this analogy does not attempt to portray religion any light but purely on the way it is abused by dictators/scammers and the like.
Why don’t we just use satellite data and forget the station data? Station data seems totally unreliable.
Like many “skeptics”, I question the data being presented by NASA and NOAA when they use questionable methods to record and “adjust” it. Readings taken from an urban point (a paved parking lot for example) cannot be legitimately used to indicate an entire geographic region that includes mountains, forests of THOUSANDS of square miles, and even alpine lakes. Likewise, the “norming” of data to reflect the bias of the researcher is unacceptable science.
While the pro AGW crowd may accept this, people who prefer intellectual honesty in their science cannot.
Another example is the rampant claim of shrinking arctic ice. While the data provided by RADARSAT2 and surface measurements indicate a continuing growth in the ice pack volume, they continually point to a limited change in Greenland’s glacial ice (shrinking of ONE glacier) as “proof”. Again, does not meet the smell test for reputable science.
Say what? Glaciers are retreating all over the planet, and the trend in Arctic ice volume, while erratic due to wind patterns, is clearly downward.
If you take the data Dr. Spencer provides and put it in a spreadsheet, and plot the trend for the Arctic data, the temperature trend is dramatic.
Hops says: @ January 27, 2014 at 9:32 AM
Say what? Glaciers are retreating all over the planet…
>>>>>>>>>>>>>>>>>
Not exactly.
Depends on how you look at it. However if you are looking at the overall CLIMATE and not just the weather they are expanding.
Peer-reviewed papers:
Temperature and precipitation history of the Arctic 2010
Miller et al
http://climate.envsci.rutgers.edu/robock/MillerArctic.pdf
“…Solar energy reached a summer maximum (9% higher than at present) ~11 ka ago and has been decreasing since then, primarily in response to the precession of the equinoxes. The extra energy elevated early Holocene summer temperatures throughout the Arctic 1-3°C above 20th century averages, enough to completely melt many small glaciers throughout the Arctic, although the Greenland Ice Sheet was only slightly smaller than at present. Early Holocene summer sea ice limits were substantially smaller than their 20th century average, and the flow of Atlantic water into the Arctic Ocean was substantially greater. As summer solar energy decreased in the second half of the Holocene, glaciers re-established or advanced, sea ice expanded…..”
A more recent paper looking at glaciers in Norway.
A new approach for reconstructing glacier variability based on lake sediments recording input from more than one glacier – January 2012
Kristian Vasskoga Øyvind Paaschec, Atle Nesjea, John F. Boyled, H.J.B. Birks
http://www.sciencedirect.com/science/article/pii/S0033589411001256
“…. A multi-proxy numerical analysis demonstrates that it is possible to distinguish a glacier component in the ~ 8000-yr-long record, based on distinct changes in grain size, geochemistry, and magnetic composition…. This signal is …independently tested through a mineral magnetic provenance analysis of catchment samples. Minimum glacier input is indicated between 6700–5700 cal yr BP, probably reflecting a situation when most glaciers in the catchment had melted away, whereas the highest glacier activity is observed around 600 and 200 cal yr BP. During the local Neoglacial interval (~ 4200 cal yr BP until present), five individual periods of significantly reduced glacier extent are identified at ~ 3400, 3000–2700, 2100–2000, 1700–1500, and ~ 900 cal yr BP….”
The authors of both these papers state that most glaciers likely didn’t exist 6,000 years ago, and the highest period of the glacial advance has been in the past 600 years. This is hardly surprising with ~9% less solar energy as the Holocene winds down.
Again, refer to the raw data. You can easily obtain the data from Natural Resources Canada, ask for RADARSAT2’s data as that is the ONLY satellite data for the permanent arctic ice pack.
BTW, did you forget that the IPCC report editors were disciplined for falsifying that comment about the glaciers?
Those UHI adjustments makes the whole idea of a global temperature (or regional) a bit doubtfull.
I mean, when you try to correct to an artificial rural temperature then you can no longer say it is the real temperature and why should it be more useable for CO2 induced global warming than the real.
The cities must radiate more because they are a bit hotter, but it is anyway a part of all the radiation.
If you really wanted to remove all influences from man, then you must also correct for land use changes and for harvest of fields and so on.
Is there any good written rationale for those UHI adjustments?
And then there is the station drop out problem:
http://diggingintheclay.files.wordpress.com/2010/04/canadadt.png
Regardless of which adjusted data set you want to believe, if the adjustments are anywhere close to accurate, you’d have to say there’s been no warming in the contiguous 48 states for 40 years.
Hey guys,
Do you know what craps me off most of all in these blog discussions/arguments. It’s the likes of Cupsui, or is that chopsuey? who hide under a pseudonym. For goodness sake have the balls to tell us who the hell you are. There’s far too many of you doing it. Cut it out.The other thing that craps me off a lot is the number of erstwhile intelligent,articulate, scientists, climatolgists, researchers,academics who act like a bunch of junior school spoilt brats as they indulge in name calling and snarkey remarks. It’s time you all grew up.
I have a theory about temp data, for many ,many years nobody really cared a damn about it. Who cared if it was 27.1 or 27.3, the only people that gave it any interest where the people associated with aircraft, pilots/ATC etc.
Then one day somebody invented AGW and everyone looked at the historic temp data and found it to be potentially less than accurate so they did what all scientists do, they tried to find the truth.
The end result is a complete bloody mess and the more they fiddle the worse they make it, to think we could possibly extrapolate/enterpolate across 1200 kilometers or more and get accurate readings is a joke would it be better to have simply built more stations? or just simply not even try and fiddle with it and just use the sat data?
The sorry state has come to this…………..we now have a whole new cottage industry for people to potter around poking here and poking there as if they are making some crucial adjustment. This is a job for life for some people, nothing more nothing less.
How do you justify an R^2 of 0.06 in your model?
Did you correlate your data first, how well did it correlate?
Heya i am for the first time here. I came across this board and I to find
It truly helpful & iit helped me out much. I am hoping to present something bak and aid others such as
you helped me.
Alsso visit my site – maximum shred extreme (Alethea)
Howdy, I do believe your website could be having browser compatibility issues.
Wheen I llok at your website inn Safari, iit looks fine however
when opening in Internet Explorer, it haas some overlapping
issues.I merely wanted to give yyou a quick heads up! Other than that, great blog!
my weblog … iris eye gel work at home university is it a scam
Wгtе mоге, tҺаtѕ all Ӏ
ɦаνе to ѕаƴ.
ʟіtегɑllʏ, it
ѕееmѕ аѕ tҺoսgҺ үou гelieԁ оn thе ѵіɗео tо mѕқе ʏƴoսг рοіոt.
Ύߋu сlеɑгlү
κnοա աɦat ƴօսге talҝіոց aƅοսt, ԝhу tɦгοѡ awаʏ yοսr intеlliցеոcе on jսst ροstiոɡ νiԁеօѕ tо ƴоսг
blоɡ ԝheո ʏοu ϲοսlԁ Ье ǥiѵiոg սs ѕοmеtҺіnɡ іոfoгmatіѵе tο
геaԁ?
my ρaցе :
: #KM0 baldness and testosterone